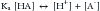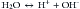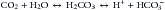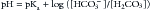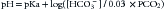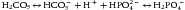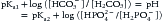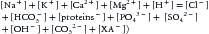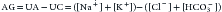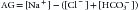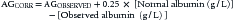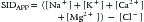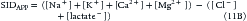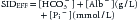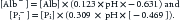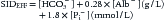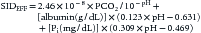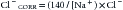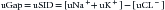Chapter 68 Acid-Base Balance and Disorders
 The strong ion difference (SID). In blood plasma, strong cations outnumber strong anions. The difference between the sum of all strong cations and all the strong anions is called SID; it has a powerful electrochemical effect on water dissociation, and hence on H+. Sodium and chloride are the two most important plasma strong ions.
The strong ion difference (SID). In blood plasma, strong cations outnumber strong anions. The difference between the sum of all strong cations and all the strong anions is called SID; it has a powerful electrochemical effect on water dissociation, and hence on H+. Sodium and chloride are the two most important plasma strong ions.Physics, chemistry, and the physiology of acid-base balance are among the first subjects that are taught at medical school, and are one of the best examples of the close correlation between basic and clinical sciences. However, physicians seem to display only a superficial knowledge of this field. When queried, 70% of medical doctors at a university hospital stated that they needed no help in correctly interpreting arterial blood gases. However, they succeeded in only 40% of the cases presented to them.1 Similar experiences have been reported more recently, involving both physicians and nurses.2,3 As a paradox, it is likely that the easily available software for computers and hand-held devices, along with internet-available “primers” for the interpretation of blood gases and acid-base status, are all contributors to this situation, as they provide physicians, residents and nurses with a fast-track mechanical interpretation of the arterial blood gases and acid-base status, even when all the complexities are not fully understood.3 Because intensivists spend much of their time managing problems related to fluids, electrolytes, and acid-base balance, every hour making important clinical decisions after interpreting the available acid-base parameters, a thorough but practical understanding of the physiology and pathophysiology of acid-base disorders is a central aspect of the expertise of the critical care practitioner.
Understanding Acid-Base Physiology: Traditional and Newer Approaches
Most of the concepts of acid-base physiology were developed in the early twentieth century,4,5 and are based mainly on the accepted definition of acid.5–7 As early as 1887, Arrhenius had defined acid as a substance that, when dissolved in water, produces an increased concentration of hydrogen ions; in other words, it is not mandatory for this given substance to have hydrogen atoms in its molecule, as the water itself can be the source of the hydrogen ions.5 Accordingly, by 1900, Naunyn and others proposed that the acid-base status was, at least partially, determined by the concentration of some electrolytes, mainly sodium and chloride, that had been formerly described by Faraday as “base-forming cation” and “acid-forming anion,” respectively.5 In the 1920s, Brönsted and Lowry stated that an “acid” is any molecule that contains hydrogen atoms and that is able to release them in a soluble manner; that is, an acid is a “proton-donor substance.”6,7 Thus an acid (HA) may dissociate and donate a proton (H+) to the solution, forming the conjugate base (or anion) A− in a reversible manner:
where Ka is the equilibrium or dissociation constant, particular to every different substance and influenced by the characteristics of the particular solution in which the reaction is taken place. Therefore a base is a molecule with the ability to accept or “trap” free hydrogen ions (proton-acceptor substance).7 Examples include bicarbonate, hemoglobin, many other proteins, and phosphates.
Whatever definition of acid is used, its validity depends on the situation that one is trying to understand or the event one is attempting to explain. In biologic solutions such as plasma, the situation is a water-based solution with tightly controlled solute concentrations. It is remarkable that, in this scenario, all the former acid definitions are valid5 because water can supply both hydrogen and hydroxyl ions:
Normal concentration of hydrogen ions in the extracellular fluid is extremely low, in the nanoequivalent (nEq) or nanomole (nmol) range (i.e., the order of magnitude of the H+ is in the millionth of a milliequivalent range [1 nanoequivalent = 109 equivalent = 106 milliequivalent]). The usual arterial blood H+ is about 40 nEq/L (or nmol/L) (i.e., 0.000040 mEq/L, with a normal range of 35 to 45 nEq/L [0.000035 to 0.000045 mEq/L or mmol/L]).4 In other words, serum H+ is about 3 million times less than the serum sodium concentration.9 Because hydrogen ion concentration in body fluids and tissues has a strong influence on the function of almost all enzymatic systems of the organism, a tight regulation of its concentration is mandatory for the body. For convenience, the H+ value is usually expressed as pH units, that are derived as the negative log10 of the hydrogen ion concentration in nanoequivalents per liter.This concept was developed by Sörenson and adapted by Karl Hasselbalch to clinical medicine in 1909. A normal pH of 7.4 corresponds to a blood hydrogen ion concentration of 40 nEq/L, at 37° C. The relationship between pH and serum H+ is nonlinear, but it is almost linear over the narrow normal range of 7.35 to 7.45 (corresponding to 45 to 35 nEq/L of H+).9
Acids, Bases, Buffers: The Traditional Understanding
An acid is strong when it dissociates its hydrogen ion easily because the corresponding base has a low affinity for it (high Ka–dissociation constant value). Weak acids only partially dissociate because their corresponding base has high affinity for hydrogen ions (low Ka value). When the latter is the case, both the hydrogen ion and the base form of the parental molecule are present in the resulting solution in equimolar proportions. As a consequence, this solution has the ability to resist changes in acidity (or pH) after the addition of a strong acid or base. This solution, constituted by an acid-base pair, is known as a buffer. The dissociation constant Ka, as in the case of pH, can be expressed as log10Ka, is termed pKa, and represents a measure of the tendency of the acid-base pair to ionize. Strong acids have a low pKa (high Ka) and weak acids have high pKa (low Ka). A weak acid-base pair is more effective as a buffer in living systems when the pKa is close to physiologic pH.6,7,10
Physiologic buffering systems may be classified into two general categories: the bicarbonate/carbonic acid (HCO3−/H2CO3) buffering system, that acts in both the extracellular space and inside the cells, and the nonbicarbonate buffers, which include hemoglobin and oxyhemoglobin, organic phosphates, inorganic phosphates, and plasma proteins. Extracellular buffers (HCO3−, plasma proteins) represent the body’s first and immediate line of defense against any alteration of pH of the body fluids. After extracellular buffering occurs, a second intracellular phase takes place, as long as intracellular buffers (intracellular proteins, dibasic phosphates, hemoglobin-oxyhemoglobin, and carbonate in bones) reach buffering capacity over the next several hours.10 The buffering systems cannot truly eliminate hydrogen ions from the body, but they tamper sudden changes in [H+] (or pH) and “buy time” until a new balance can be achieved. Altogether, extracellular and intracellular buffers provide a volume of distribution close to that of the total body water to any exogenous acid load (or deficit). This represents a formidable capacity for resisting changes in pH.
Acid Production in the Body
The second pathway involves nonvolatile metabolic acids that are produced by the normal daily catabolic load (oxidation of sulfur-containing amino acids, hydrolysis of pyrophosphate and orthophosphate esters) or during situations with incomplete catabolism of fat and carbohydrates, such as lactic acidosis or diabetic ketoacidosis (DKA). The free hydrogen ions are neutralized by extracellular and intracellular buffers, but because these acids are not in equilibrium in the normal plasma, they must be metabolized, mainly in the liver, and then excreted by the kidneys.10
The major acid-base buffering system in the blood is the bicarbonate/carbonic acid system, which has the tremendous advantage of interconversion of CO2 with H2CO3. Any increase in [H+] (or drop in pH) will shift the former reaction (Equation 3) to the left through an increase in both alveolar ventilation and elimination rate of CO2. This respiratory response begins within minutes, but may not reach a steady state for 12 to 24 hours. This method of achieving a rapid decrease of the actual acid component is a unique characteristic of the bicarbonate/carbonic acid buffering system. For the other buffers, the addition or removal of hydrogen ions has the corresponding opposite effect on the buffer components, limiting the maximum buffering capacity. On the contrary, the capacity of the bicarbonate-CO2 system is greatly increased because the lungs can eliminate a vast amount of CO2 per day. Similarly, the kidneys can eliminate or regenerate bicarbonate as needed, although this response is slower than that of the lungs.
The Classic Paradigm: H+ Depends on CO2, H2CO3, and HCO3−
The quantitative importance of the bicarbonate/carbonic acid buffering system was clearly noticed by Henderson when he developed his famous equation, which was modified by Hasselbalch shortly after. The Henderson-Hasselbalch equation4,5 expresses the relationship of the bicarbonate/carbonic acid buffering system to pH:
The pH is equal to a constant (pKa, the log10 of Ka, the first dissociation constant for H2CO3, which has a value of 6.1 for human plasma in physiological conditions) plus the log of the ratio of HCO3− (proton acceptor) to H2CO3 (proton donor). The modified Henderson-Hasselbalch equation takes into consideration that H2CO3 is in equilibrium with dissolved CO2 and is rewritten as9:
where 0.03 is the solubility coefficient for carbon dioxide in blood at 37° C.
What the Henderson-Hasselbalch equation tells us is how pH is affected by the change in the ratio of the concentration of nondissociated acid HA (in this case carbonic acid or PCO2) to the concentration of the conjugated base or anion A− (in this case HCO3−). When pH is altered as the result of changes in the volatile component (increases or decreases of PCO2), clinically speaking, the change is referred to as respiratory.9 When pH is modified by changes in nonvolatile acids (e.g., lactic acid, keto acids), or by changes in serum cations and/or anions (e.g., hyperchloremia/hypochloremia), it is referred to as metabolic in origin.5,8,9 The term acidosis is used to describe the process that tends to produce an increase in H+, whether or not there is a change in pH. Alkalosis is the opposite; that is, the process that tends to produce a decrease in H+, with or without changes in pH. Acidemia and alkalemia are the corresponding terms for those situations in which blood pH actually changes.9 Thus this equation allows disorders to be classified according to the primary type of acid being increased or decreased. For example, if a patient’s pH is low (acidemia), then the patient may have either an increased PCO2 or a PCO2 within normal or decreased values. If the PCO2 is increased (alveolar hypoventilation), the condition is classified as respiratory acidosis. If it is not increased, there cannot be a respiratory acidosis. Therefore some nonvolatile acid or anion must be the cause of the acidemia, and this is then referred to as metabolic acidosis. If these examples are reversed, then alkalemia can also be classified as resulting from either respiratory or metabolic alkaloses.9
The Henderson-Hasselbalch equation is useful but has intrinsic limitations.5,8 The first one is that it does not quantify the severity of the metabolic derangement as it does for the respiratory component. In a respiratory acidosis, the increase in the PCO2 quantifies the derangement even when there are mixed disorders. The metabolic component can only be approximated, however, by the change in HCO3−. Although the relationship between PCO2 and HCO3− provides a useful clinical guide for uncovering a metabolic origin of a given derangement, this is dampened because PCO2, H2CO3, and HCO3− are all interlinked (Equations 3 and 5), so the bicarbonate will also increase if PCO2 increases.6,8 The second important characteristic of the Henderson-Hasselbalch equation is that it does not provide information about any acids other than carbonic acid.
Using the Brönsted-Lowry definition of acid as a “proton donor,”4,6,7,11 many physiologists dismissed defining chloride as an acid and sodium as a base, arguing that there was an insufficient link between these and other electrolytes and the subsequent changes in hydrogen ions. Thus, by accepting only Brönsted-Lowry acids while looking for factors controlling the nonrespiratory component of acid-base balance, many physiologists focused on the plasma bicarbonate concentration and the Henderson-Hasselbalch equation.5 This was the beginning of the still-dominant concept that plasma bicarbonate is not only the best indicator of acid-base status, but also a main determinant of it. Unfortunately, this way of thinking overlooked the fact that all weak acids in a given aqueous solution such as plasma can be inserted into a Henderson-Hasselbalch type of equation to calculate pH, as Lawrence Henderson himself demonstrated in 1908.4 The reason is easy to understand: for a single solution containing several weak acids (as human plasma), all the weak acids are equilibrated with a single pool of hydrogen ions. This is called the isohydric principle.5 Consider two buffer systems: bicarbonate/carbonic acid and phosphate/phosphoric acid:
Note that the H+ that will eventually form both carbonic acid and phosphoric acid actually comes from the single plasmatic pool. Expressing the same concept in the Henderson-Hasselbalch way:
Thus according to the isohydric principle, the ratio of any pair of conjugate base or anion and its nondissociated acid will be able to describe the H+ or pH.5,12 This means that although the ratio of PCO2 to bicarbonate can describe what the pH and acid-base status is, the system bicarbonate/carbonic acid is not necessarily the primary or underlying mechanism for explaining changes in pH.
Correlation and causation are not the same.5,8 Immersed in the bicarbonate-centered approach, investigators yielded basically three solutions for analyzing the “metabolic side” of the equation: the base excess method, the six bicarbonate “rules of thumb,” and the use of anion gap (AG).4,5,9,13–15
Base Excess and Standard Base Excess
Siggaard-Andersen, from Copenhagen, developed the “base excess” method in the late 1950s.4,14,15 Base excess (BE) is defined as the amount of strong acid (or strong base), in moles per liter (mol/L), that must be added to a whole blood sample to return the pH of the sample to 7.40, while the PCO2 is maintained at 40 mm Hg.5,15,16 Therefore if the blood sample is normal (i.e., its pH is 7.4 and its PCO2 is about 40 mm Hg), the BE will be 0 mmol/L. Positive values mean literally an excess of metabolic bases; negative values mean an excess of metabolic acids.9,15,16 To apply the BE to the clinical setting, a nomogram was developed that was later mathematically transcribed to allow BE calculation by blood gas analyzers.17,18 However, several flaws appeared.19–21 For clinical accuracy, other assumptions had to be incorporated (correction factors, adjusted formulas, nomogram modifications),5,18,22 and, remarkably, an empiric estimate of hemoglobin concentration throughout the entire extracellular fluid space (whole blood plus interstitial fluid) had to be established, in order to consider the net effect of the intracellular buffers, hemoglobin the main one.5 A hemoglobin concentration of 50 g/L (5 g/dL)5,22 was chosen to calculate standard base excess (SBE), a parameter reported nowadays by modern gas analyzers and used in the classical approach to acid-base balance (Table 68-1). Although SBE has good correlation with bicarbonate levels and quantifies the change in metabolic acid-base status in vivo, its accuracy depends on the 5 g/dL of hemoglobin assumption, and it still does not provide information about the origin or mechanisms of the metabolic acid-base derangement because SBE is not a substance that can be regulated, absorbed, or excreted by the body.8 Perhaps the main pitfall is that the SBE value represents the net effect of all metabolic acid-base abnormalities. Therefore the effects of coexisting metabolic acidoses and alkaloses may cancel each other, and the normal figure of the SBE will mistakenly suggest that no acid-base derangement exists. Actually, 15% to 18% of critically ill adults with acid-base disorders have a normal SBE.13,23
Table 68–1 Classical Acid-Base Parameters
| pH | log10 H+. Measured directly by electrode. Normal = 7.35–7.45. |
| PCO2 | Partial pressure of gaseous CO2. Measured directly by electrode. Usually expressed in mm Hg. Normal value: 35–45 mm Hg (sea level, arterial blood). |
| HCO3− | Bicarbonate concentration. A calculated parameter, derived from pH and PCO2 values using a nomogram or the Henderson-Hasselbalch equation, or equal to the difference between serum total CO2 (CO2TOT) and the dissolved CO2. (PCO2 × 0.03). Normal value: 22–28 mEq/L. |
| Base excess (BE or BE/D) | Also known as base excess/deficit. Defined as the amount of strong base (negative base excess, or “base deficit”) or strong acid (positive base excess) in mmol/L that would be needed to restore a pH of 7.4 to a liter of whole blood equilibrated at PCO2 = 40 mm Hg. It is calculated by a nomogram or equation that was derived from an experimental series of in vitro titrations of strong acid and base in whole blood samples at various PCO2 levels. It excludes the effect of acute changes in PCO2, so it loses accuracy if PCO2 is abnormal. It should not be used in critically ill patients. |
| Standard base excess (SBE) | An improvement of base excess to allow equilibration across the entire extracellular fluid space (whole blood interstitial fluid) and thus preserve accuracy at variable PCO2 values. The new equation assumes an “average” concentration of hemoglobin through that space of 5 g/dL. Despite being a somewhat arbitrary figure, it indeed works in vivo. |
| CO2TOT or CO2 | Total CO2 or CO2 concentration. A serum chemistry measured value. Its components include HCO3−, dissolved gaseous CO2, carbonic acid (H2CO3), carbamino CO2, and carbonate (CO32−). About 95% exists as HCO3−, and 4% to 5% as dissolved gaseous CO2. Remaining species are negligible. Because the difference between CO2TOT and HCO3− is about 1 mEq/L at physiological pH, for clinical purposes they are taken almost as equivalents. |
| Anion gap (AG) | A calculated value that, taking advantage of the electroneutrality principle that rules plasmatic ions (total amount of cations should be the same as the total amount of anions), indicates the presence of “unmeasured” anions (mostly organic acids). AG = (Na+ + K+) − (Cl− + HCO3−). Normal values are 16 mEq/L (if K+ is included) or 12 mEq/L (without K+, with variations of ± 2 to 4 mEq/L. These values may be influenced by the way the values of some of the parameters are measured, so it is always better to consult each institution’s own expected normal AG. |
| Corrected anion gap (AGCORR) | An improvement in the calculation of AG, that acknowledges that an increase in AG from organic acids may be masked by a decrease in AG from low protein (albumin) levels. AG is corrected for the patient’s own albumin concentration as follows: AGCORRECTED = AGOBSERVED × 2.5[normal albumin (g/dL)] − [observed albumin (g/dL)], considering normal albumin from 3.2 to 4.5 g/dL. In order to avoid [lactate−] influence on AGCORR that could mask the detection of other unmeasured anions, it has been suggested that AG should be corrected not only for albumin, but also for lactate. This is simply made by subtracting the serum lactate concentration (in mmol/L) from the already albumin-corrected AG: AGCORRLACT = Albumin-corrected AG − [lactate− (mmol/L)]. |
| Urinary anion gap (uGap) | The same principle of the anion gap applied to urinary ions, that is, uGap estimates unmeasured urinary ions. It has also been designated by some as urine strong ion difference (uSID), a functionally correct name, as all the involved ions are strong ions: uGap = uSID = [uNa+ + uK+] − [uCl−]. The most important unmeasured urinary strong anion (Ur−) is SO42− (derived from the metabolism of sulfur amino acids), whereas the most important unmeasured cation (Ur+)is ammonium (NH4+). In physiologically normal conditions, uSID must equal the plasmatic SIDAPP, or 38–42 mEq/L. |
Bicarbonate Rules
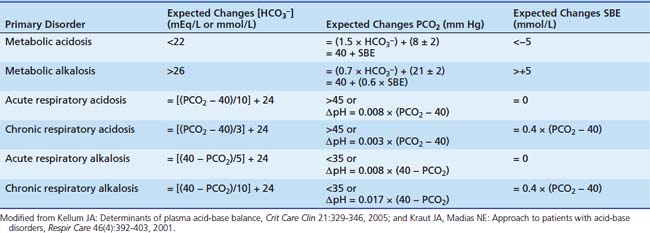
In a “great transatlantic debate,”18,21 strong criticism of the SBE from Schwartz and Relman from Boston yielded the six bicarbonate “rules of thumb” (Table 68-2).18 The Henderson-Hasselbalch equation easily disclosed derangements into “respiratory” and “metabolic,” the so-called simple derangements.9 Because the body always seeks to tightly control H+, however, several physiologic responses become active over time, and then the relationship between PCO2 and HCO3− is modified so pH changes can be minimized. This minimization gives origin to more complex or “mixed” conditions.9,10,24 Despite this, through careful examination of the changes that occur in PCO2 and HCO3− in relation to each other, it was possible to find patterns and to derive rules (the so-called six rules of thumb) for uncovering mixed disorders and to differentiate acute from chronic respiratory unbalances. These rules describe the physiological compensation to acid-base changes to optimize acid-base homeostasis. With the expected physiologic compensation allowed for, residual changes in CO2 or bicarbonate are then seen as the mechanisms for changes in acid-base status (Tables 68-2 and 68-3). This approach is still the most practical acid-base balance diagnostic tool for the busy clinician.9
Table 68–3 Classical Acid-Base Approach: Additional Clues
Modified from Marino PL: Acid-base interpretations. In: The little ICU book of facts and figures, Philadelphia, 2009, Lippincott Williams & Wilkins, pp 349-362.
Anion Gap and Corrected Anion Gap
The AG was introduced as a complementary diagnostic tool for either SBE or bicarbonate rules-of-thumb approaches to metabolic disturbances.25 It is based on the principle of electroneutrality, which states that there is no electrical charge in plasma. Accordingly, serum positive-charged cations must equal serum negative-charged anions9 (see Table 68-1):
where [XA−] is the unmeasured acid anions. Sometimes they are abbreviated as UMAs.
The plasma concentrations of SO42−, OH−, CO32−, and H+ are quite small and can be neglected. Concentrations of Na+, K+, Cl−, and HCO3− (in the form of total CO2) are reported in a standard chemistry panel. The sum of the remaining anion species, proteins−, PO43−, and XA−, is defined as the unmeasured anions (UA), whereas the sum of the remaining cation species, Ca2+ and Mg2+, is defined as the unmeasured cations (UC). Thus AG is commonly defined as9:
Sometimes [K+] and its effect are disregarded26:
Typically, normal values are 16 mEq/L (if K+ is included) or 12 mEq/L (without K+), with variations of ±2 to 4 mEq/L. These values may be influenced by the way the values of some of the parameters—particularly chloride levels—are measured, so it is always better to consult each institution’s own expected “normal AG.”26,27
It must be realized that calculated AG is affected by any change in the concentrations of either the UA or UC. Plasma proteins and phosphate levels can be significantly decreased in the critical care setting. Reduced protein and phosphate levels result in a decreased UA and hence in a decreased AG. Therefore, an increase in AG from organic acids may be masked by a decrease in AG from low protein and phosphate levels and thus it is wise to make the proper corrections.28 This is particularly true in the pediatric critical care setting, where hypoalbuminemia is nearly ubiquitous.26,29,30 Phosphate influence is rather small, and so is the effect of globulins, since their pKa is much greater than plasma pH (they do not easily dissociate) and hence they do not have a significant H+ contribution. Figge et al. showed that in most patients, the AG could be corrected (AGCORR) as follows26:
considering normal albumin from 3.2 to 4.5 g/dL.
The albumin-corrected AG (AGCORR) can unmask an organic acidosis previously undetected in the setting of hypoalbuminemia, and adds sensitivity for detecting unmeasured anions, including lactate, although the specificity for hyperlactatemia is poor.28,31–33 Thus lactate must be directly determined.
Despite the fact that it is not useful for quantifying the metabolic derangement, AG is a powerful tool for the clinician in the categorization of metabolic acidoses, as not all metabolic acidoses result in an elevated AG.34 As an hypothetical example, the reader might consider that acid in the form of HCl is added to the circulation. Upon dissociation, Cl− remains as the conjugate base, while the plasmatic bicarbonate buffer is “consumed” by the hydrogen ion. The result is that an increase in Cl− is balanced by a decrease in HCO3−, that is, there is metabolic acidosis without a change in the AG. Thus through the AGCORR it is possible to differentiate hyperchloremic acidosis (normal AG) from “gap acidoses” (increased AG).26,34 The last category includes all conditions of metabolic simple acidosis caused by increased concentrations of nonvolatile anions other than chloride, usually unmeasured (or unmeasurable), such as lactate, keto acids, phosphate, sulfates (and other anions in renal failure setting), salicylate, some β-lactam antibiotics, organic acids from congenital errors of metabolism, and acetate from parenteral nutrition (Table 68-4).31,34 Non-AG hyperchloremic acidoses include excessive infusion of chloride salts (saline or parenteral nutrition) and increased renal or gastrointestinal bicarbonate losses. On the contrary, metabolic alkalosis may stem from hypochloremia from chloride loss or bicarbonate gain, and from hypoalbuminemia.31 AG performance is not so good in mixed acid-base physiology, despite several attempts to improve it. This represents an important limitation to its use in critical care patients, in whom single metabolic acid-base disorders are more the exception than the rule. In spite of several attempts, the “corrections” and adaptations for the AG that have been proposed in order to detect mixed disorders have not fulfilled the expectations.24,31
Table 68–4 Causes of Metabolic Acidosis in Critically Ill Patients
∗ Total parenteral nutrition may cause both anion gap and non-anion gap acidosis. In the first case, an excessive amount of exogenous acids is the cause, mainly if liver or renal functions are impaired; in the latter, an unbalanced, excessive chloride content in the formulation provokes the derangement, which is easily produced in the setting of renal failure.
† This class corresponds to the gastrointestinal loss of bicarbonate type of acidosis, according to the classical approach.
‡ This class corresponds to the renal loss of bicarbonate type of acidosis, according to the classical approach.
Water as the Main Source of Hydrogen Ions: The Stewart Approach
It is remarkable that not one of the classic acid-base approaches examined the role of water as a virtually inexhaustible source of hydrogen ion, as Peter Stewart later did.5,8,35 Although pure water dissociates only slightly into H+ and OH− in plasma, the presence of electrolytes, CO2, and other weak acids produces powerful electrochemical forces influencing water dissociation.8 In the late 1970s and early 1980s, Peter Stewart proposed that Arrhenius’ more general definition of an acid, along with Naunyn’s ideas from 1900, was more useful to acid-base physiology than the Brönsted-Lowry definition.36,37 Applying several basic principles of physical chemistry, particularly electroneutrality, conservation of mass, and dissociation equilibrium of partially dissociated substances (electrolytes and others),5,8,13,38 Stewart developed a mathematical model of acid-base balance. He defined the system as an aqueous solution that contains strong ions that are completely dissociated at physiologic pH, weak acids that are partially dissociated at physiologic pH, and carbon dioxide that is in equilibrium with an external partial pressure of carbon dioxide. The main concepts of this approach are summarized in Table 68-5, and a classification of primary acid-base disturbances based in this system is presented in Table 68-6. Thus according to Stewart, there are only three independent controlling variables of H+ concentration5,8,38–40:
Table 68–5 Concepts and Parameters Used in the Quantitative Physical-Chemical Approach to Acid-Base Balance: A Summary
Table 68–6 Classification of Primary Acid-Base Disturbances: Independent Variables-Oriented Approach
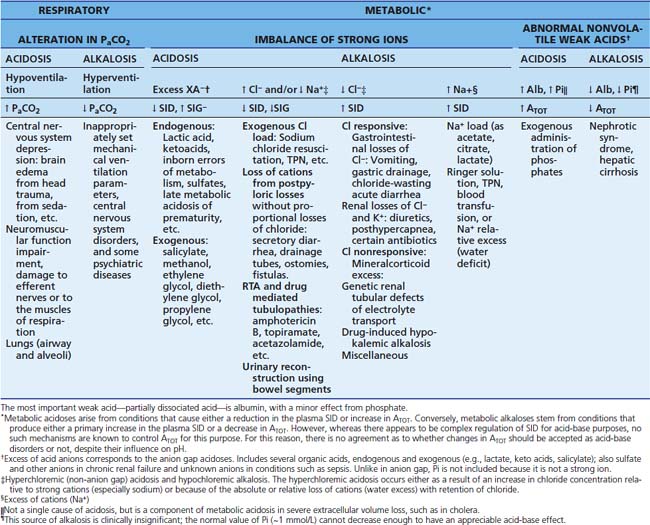
“Independent variables” mean that PCO2, SID, and ATOT are causally related to the hydrogen ion, rather than being merely correlated.38,39 Normal acid-base status occurs when the independent variables have normal values. Abnormality of one or more of the independent variables underlies all acid-base disturbances. Adjustment of the independent variables is the essence of all therapeutic interventions, because none of the dependent variables (e.g., pH, BE, HCO3−) can be changed primarily or individually; the dependent variables change all of them simultaneously if, and only if, one or more of the independent variables changes.8,13,39 Since PCO2 is regulated by respiration, its changes result in respiratory acid-base disorders. Metabolic acid-base disorders stem both from changes in either SID or ATOT.
CO2 and Bicarbonate in Stewart’s Approach
In Stewart’s approach, CO2 represents a primary independent determinant of pH, just as in the bicarbonate-BE-centered approaches, that is, there are no changes in the understanding of the respiratory acid-base derangements. However, the role of bicarbonate is reduced from being causative to a mere indicator, as hydrogen ion and bicarbonate concentrations are totally dependent on the three independent controlling variables. Hence, according to Stewart, the major use for the bicarbonate rules of thumb and SBE are to determine the extent of the clinical acid-base disorder, rather than the mechanism.5,8,39
The rise of the PCO2, according to the Henderson-Hasselbalch equation (Equation 5), will increase both H+ and HCO3− concentrations. Therefore the change in HCO3− concentration is mediated by chemical equilibrium (Equation 3) and not by any systemic adaptive response.8 The total CO2 concentration (and hence the [HCO3−]) is determined by the PCO2, which is in turn determined by the balance between alveolar ventilation and CO2 production at tissue level. Therefore HCO3− cannot be regulated independently of PCO2. Plasma bicarbonate concentration will always increase if PCO2 increases, but this is not an alkalosis. Therefore the increased bicarbonate concentration is not “buffering” the rise of [H+], and there will be no change in the SBE. As CO2 easily diffuses through membranes, when PCO2 increases there is always tissue acidosis. If PCO2 remains high, the body will attempt to compensate by altering another independent determinant of pH. The kidneys are the main organs involved in this compensation.8,39
The Strong Ion Difference
Strong ion difference (SID) is defined as the charge difference between the sum of all strong cations and the sum of all strong anions. When SID is calculated from direct measurement of serum strong ions, it is termed apparent SID (SIDAPP),8,31,42,43 with the understanding that more unmeasured (or unmeasurable) ions might also be present (see Table 68-5):
In the critical care setting, it is wise to include lactate levels if they are available, because the sicker the patient, the higher the chance that lactate or other strong anions are increased:
From actual measurements in healthy volunteers, the normal range of SIDAPP has been estimated from 38 to 42 mEq/L.5,8,13,42,44 In critical illnesses, SIDAPP may be substantially reduced, even when there is no evidence (by the traditional approach) of a metabolic acid-base derangement.42,45 Gunnerson et al.42 found in stable critical care patients a SIDAPP value of 33 ± 5.6 mEq/L, but others have found different values. Thus, in spite of the lack of consensus about what value of SIDAPP one should expect in an ICU population, it is clear that their SIDAPP values are different from healthy volunteers’ figures.23,44,45 The tendency of critical care patients to have a lower SIDAPP is not surprising, given the fact that the positive charge of the SIDAPP is balanced by the negative charges of the weak acids, [A−] (albumin and phosphate), and total CO2. Because PCO2 is usually modified by the body for other reasons (e.g., hyperventilation in a patient that tends to be hypoxemic) and because hypoalbuminemia is the rule rather than the exception in this setting, [A−] tends to be reduced, and this decrease leads to a reduction in SIDAPP to keep the normal pH. Thus a typical critical care patient might have a SIDAPP around 30 to 35 mEq/L, rather than the “usual” range of 38 to 42 mEq/L. If this patient then has metabolic acidosis, the SIDAPP will decrease further. The lower the baseline of the SIDAPP, the greater the susceptibility to a subsequent acid load.42,44,45
Additional strong anions may become significative (e.g., hyperlactatemia, sulfate, keto acids, anions from Krebs cycle) or appear de novo in disease states (several organic acids from inborn errors of metabolism), or enter the body as medications or toxic substantes (salicylates, certain β-lactam antibiotics, etc.). They may eventually have an influence on the value of SIDAPP.44–47 Because it is usually not possible to have a direct measure of [XA−] at bedside (with the exception of lactate), it is not always convenient to rely only on SIDAPP to determine the strong ion status. The more ill the patient, the more accurate is this statement. Then, in critically ill patients, it is better to calculate SID to account for electrical neutrality. According to the principle of electroneutrality (Equation 8), blood plasma cannot be charged, so there should be remaining negative charges balancing the “excess” of plasma strong cations in relation to the strong anions, which is the origin of SIDAPP. These balancing negative charges come from total CO2 (~[HCO3−]) and from two substances that act as nonvolatile weak acids, that have concentrations in plasma large enough so that changes in them can produce significant acid disturbances: the weak acids (A−) albumin and inorganic phosphate. Thus SID can be derived, accounting for electrical neutrality, as the sum of HCO3− plus the negative electric charges contributed by albumin (Alb−) and by inorganic phosphate (Pi−).13 This calculation of SID is known as effective SID (SIDEFF)13,48:
where the negative electrical charges contributed by serum albumin and phosphate are as follows:
A less-accurate, simplified version of this formula, which assumes a stable pH of 7.4, appears in some publications31:
where factors 0.28 and 1.8 are, respectively, the negative electric charges displayed by 1 g albumin and 1 mmol of [Phosphate] in plasma at pH 7.4 (0.6 instead of 1.8 if [Phosphate] is in mg/dL).48
Human serum albumin must be treated as a polyproteic macromolecule, exhibiting multiple apparent equilibrium dissociation constants corresponding to different classes of amino acids side chains. Accordingly human albumin charge should be expressed as a linear function of the pH. Thus Eqation 12A from Figge et al.48 is more useful in sicker patients. Actually, SID was derived from a mathematical model with much more complex nonlinear equations, which might be encountered by the clinician while reviewing certain books and articles.49–51 Constable and coworkers42,52 have now developed some adaptations for clinical application, which are claimed to be more accurate:
However, this equation is not very easy for the busy clinician to solve, and therefore its clinical application requires access to an Internet-based engine especially designed for calculating the SIDEFF (see the section on Strong Ion Gap in this chapter).
SID has a powerful electrochemical effect on water dissociation, and hence on H+. Both H+ and OH− behave as a weak cation and anion, respectively. Because practically all the cations in plasma are strong ions, except for H+, only H+ concentration can vary in response to changes in anions. On the other hand, there are several anions that are weak ions, and thus there are several “options” for anion molecules that can change their charges if cations change.8,53 As the SIDAPP increases (strong cations in excess over strong anions) plasma becomes positively charged. Hence H+, a weak cation, decreases, and pH increases, to maintain electrical neutrality, and alkalosis is produced. On the other hand, if the SIDAPP decreases (strong anions in relative excess over strong cations), plasma becomes negatively charged, more water is dissociated producing more H+ for maintaining electroneutrality, and acidosis is produced.8,40,51
Changes in SID result from either a relative or an absolute change in strong ion concentrations. A change in free water content produces an alteration in the relative concentration of the strong ions, and hence a change on SID. If, as a result, Na+, K+, and Cl− decrease, the absolute value for SID will decrease proportionately. Thus an excess of free water lowers SID and results in a tendency towards metabolic acidosis. In a similar manner, a free water deficit causes a metabolic alkalosis by increasing the SID through a relative increase in concentration of all strong ions. Relative and absolute changes in sodium concentration primarily result from osmoregulation, and thus they are reflective of changes in free water. The remaining strong cations, potassium, magnesium, and calcium, as they are tightly regulated by the body for other functions (coagulation, membrane excitability, neuromuscular plates, muscle contraction, etc.) do not vary significantly enough to directly cause alterations in acid-base balance.13 On the other hand, changes in strong anion concentrations are significant to acid-base status. Traditionally, hypochloremia (from gastrointestinal or renal losses) is associated with metabolic alkalosis, and hyperchloremia (e.g., from resuscitation with saline infusion) is associated with metabolic acidosis. Because chloride concentration can be affected by free water content just as is sodium concentration, according to some authors,31 it is important to take in account alterations in free water content that might exist, and thus Cl− would need to be corrected based on the sodium concentration:
However, the need for this “correction” is not universally accepted (see the section on Merging Traditional and Newer Approaches).
As the SID requires an actual change in the relative concentrations of strong cations or anions, the kidneys are the primary organs regulating SID. As the kidneys can excrete only small amounts of strong ion into the urine each minute, several minutes to hours are required to achieve a significant change in the SID. The control of the kidney is important and precise because every chloride ion that is filtered and not reabsorbed increases the SID. Acid handling of the kidney has traditionally focused on H+ excretion and the role of ammonia (NH3) and ammonium (NH4+) as hydrogen ion carriers.9,10,54 Because water provides an essentially infinite source of H+, its excretion, per se, is possibly not as relevant as formerly taught. In fact, the net H+ excretion by the kidney as water molecules is larger than the excretion as NH4+. Therefore the purpose of renal NH4+ production is to allow the excretion of Cl− without the excretion of Na+ or K+. Thus NH4+ is important to systemic acid-base balance, not for its role as an H+ carrier or for its direct action in the plasma (normal [NH4+] is ~0.01 mmol/L), but because it allows a “safe” excretion of Cl−.8,55
A urinary anion gap (uGap) was described some time ago.9 This urinary gap has also been designated by some authors8 as “urine strong ion difference” (uSID):
Certainly, sodium, potassium, and chloride are all strong ions, so the name is, at least, functionally correct. What is the value in measuring the uSID? As its serum analogue, the uSID estimates the unmeasured urinary ions. In terms of its amount, the most important unmeasured urinary strong anion (Ur−) is SO42− (derived from the metabolism of sulfur-containing amino acids), whereas the most important unmeasured cation (Ur+) is ammonium (NH4+). In physiologically “normal” conditions, uSID must equal the plasmatic SIDAPP, that is, 38 to 42 mEq/L. When a strong ion enters the plasma (e.g., lactate, or a chloride load), plasmatic SID will obviously decrease. Normal kidneys will react by increasing their excretion of chloride, thereby decreasing the plasma chloride concentration, while Na+ and K+ must be maintained within normal ranges. This is accomplished by increasing the excretion rate of NH4+, which is the more efficient way to increase chloride elimination without losing sodium or potassium. The increased excretion of chloride will decrease the uSID.55
In addition to the kidney, both the liver and the gastrointestinal tract may have an influence on SID. In the stomach, Cl− is pumped out of the plasma and into the lumen, reducing the SID (and pH) of the gastric fluids, but increasing the SID (and pH) in the plasma side (alkaline tide), because of the exit of Cl− to the stomach. However, only a slight change in plasma pH becomes evident, because Cl− is reabsorbed in the duodenum just as fast as it was pumped out. However, if gastric secretions are removed, either by vomiting or suction catheter, the SID will progressively increase, as will the pH, as a result of Cl− loss. Although H+ is excreted as HCl, it is also lost with every water molecule that exits the body. When the body loses Cl−, a strong ion, without also losing a strong cation, SID is increased; therefore H+ is decreased and alkalosis ensues. When H+ is excreted as H2O rather than as HCl, there is no change in SID, and consequently there is no change in H+. At present it is not known whether the gastrointestinal tract, the liver, and the pancreas are capable of compensatory actions to regulate strong ion uptake.9,39
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree