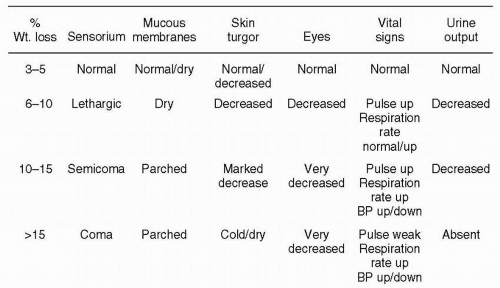
The appropriate fluid and electrolyte therapy involves the understanding of a few basic principles that can be applied systematically to calculate the requirements for an individual patient. The maintenance requirements for water are based on the studies of the energy expenditures of hospitalized children performed by Holliday and Segar. In these studies, the average energy expenditure was measured as a function of body weight. The requirement for water was then calculated by assuming that the child was consuming 2 g of protein/kg per day. A second assumption was that the products of protein metabolism were to be excreted in urine that was neither concentrated nor diluted—that is, urine with a specific gravity of 1.008 to 1.015. Given these two assumptions, the water requirement is then 1 mL/calorie expended. A simplified graph of the data on energy expenditure described in the original work of Holliday and Segar is shown in
Figure 11.1. By breaking the continuous line into three segments, it is possible to approximate the daily fluid requirements. They are as follows:
100 mL/kg for the first 10 kg of body weight
50 mL/kg for the second 10 kg of body weight
20 mL/kg for each kg above 20 kg (at least until 80 kg is reached)
This is a simple method of estimating maintenance water requirements. The daily electrolyte requirements are summarized in
Table 11.1.The fluid and electrolyte requirements of a 40-kg child are outlined in
Tables 11.2 and
11.3 Note that in 0.2% normal saline (NS) solution, the concentration of NaCl is 34 mEq/L, and if 20 mEq of KCl is added to each liter of fluid, then a correct calculation of the water requirements automatically gives the correct complement of Na, K, and Cl. This is why commercially available solutions contain 0.2% NS— because they provide adequate concentrations of electrolytes if the patient has no deficits, is stable, and requires intravenous (IV) fluids only to maintain homeostasis.
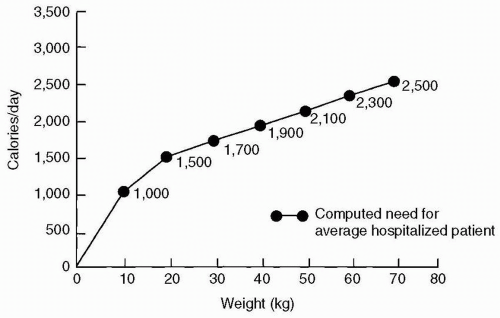
A situation that the pediatrician encounters more commonly is when the patient arrives with a history of diarrhea and dehydration and requires not only maintenance IV fluids, but also replenishment of a fluid and electrolyte deficit that has resulted from vomiting and diarrhea. Physical findings that help in estimating the severity of the deficit are shown in
Figure 11.2.
Note that
Figure 11.2 gives an estimation of the percentage of dehydration. To translate this into how much “fluid” the patient has lost, multiply the percentage loss with the body weight in kilograms. The result is the fluid deficit in liters. For example, if you evaluate a 1-year-old child who appears dehydrated, and the best estimate from the physical finding appears to be that he is 10% dehydrated and weighs 10 kg, then 0.10 (10%) × 10 kg = 1 kg (or 1 L) of fluid deficit. Remember, 1 L or 1000 mL of water weighs 1 kg!
When a fluid prescription is calculated for an individual patient, the task should involve answering three questions:
If this approach is applied consistently, fluid and electrolyte calculations will cease to be perplexing and can become routine. The major source of error when the approach is utilized is the estimation of ongoing losses. This is always a guess, and although (it is hoped) a thoughtful one, like any projection into the future, it is subject to error. Therefore, when a patient is treated with a fluid regimen and the response is not the expected one, always check to determine if a source of continuing fluid loss is present that which you did not anticipate. Or conversely, did you anticipate fluid losses that are not present?
Another calculation that is frequently utilized on board exams is that of free water deficit or free water excess. In order to solve this in a given patient, you need to know the patients weight (in kg) and the serum Na in mEq/L. Then there are 2 assumptions that are made
(Table 11.4):
The volume of distribution of Na is the extracellular fluid volume and is calculated by taking 0.6 (l/kg) × weight (kg) = volume of distribution of Na (in liters).
The number of Na ions will stay constant; the change in concentration will be the result of adding or subtracting water.
In the review exercises, three cases are presented for which you are asked to estimate fluid deficits and calculate replacement regimens.